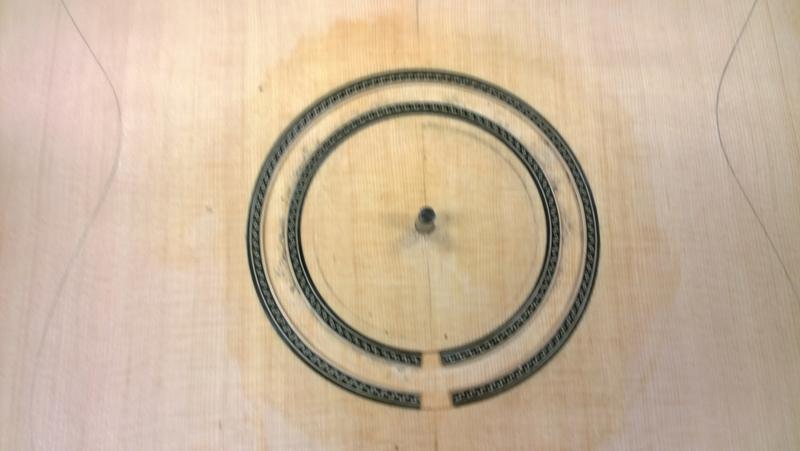
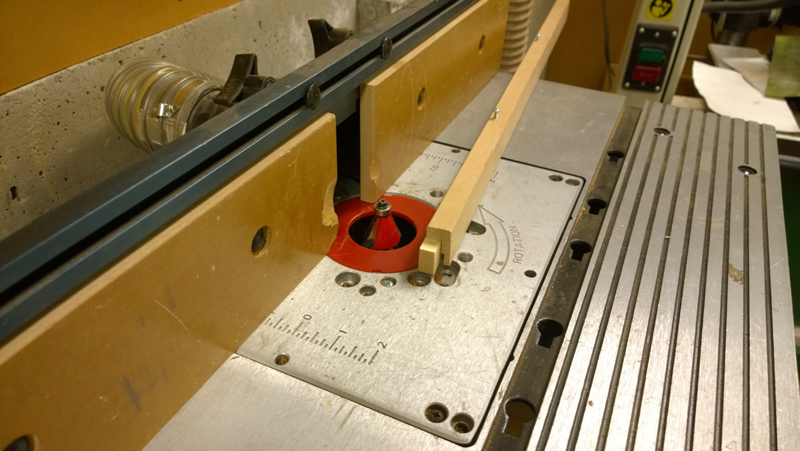
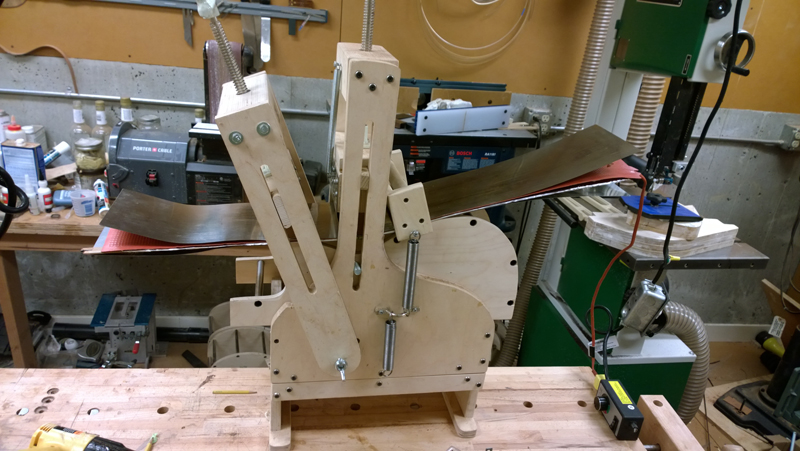
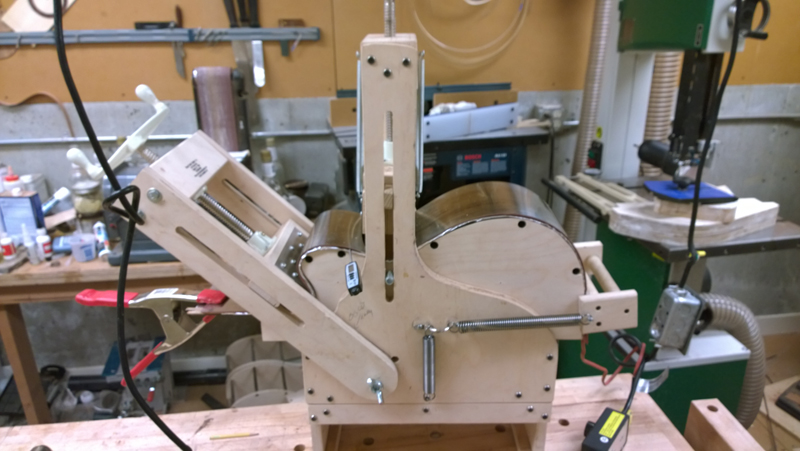
Falcate Braced Classical Guitar - Finished!
Posted: Tue Jan 27, 2015 2:01 am
I am just starting a project to build a Classical guitar with carbon fiber reinforced falcate bracing. The design of this guitar relies heavily on the guitar design concepts and the build procedures contained in the Gore\Gilet Contemporary Acoustic Design and Build books. This guitar is not being built for anyone so I do not have a time constraint nor fear of failure. I have always been a relatively lazy design engineer relying mostly on my understanding of relationships from a known point rather than starting with a clean sheet. Before starting I have read through posts on the Australian/New Zealand Luthiers Forum on the same topic http://www.anzlf.com/posting.php?mode=reply&f=33&t=3819 and a few other build threads and have reread chapter 4 in the design book. So here are my thoughts. I may be way off base but I thought I would share them. Sorry about references to specific topics in the books, but that is what this blog will be about.
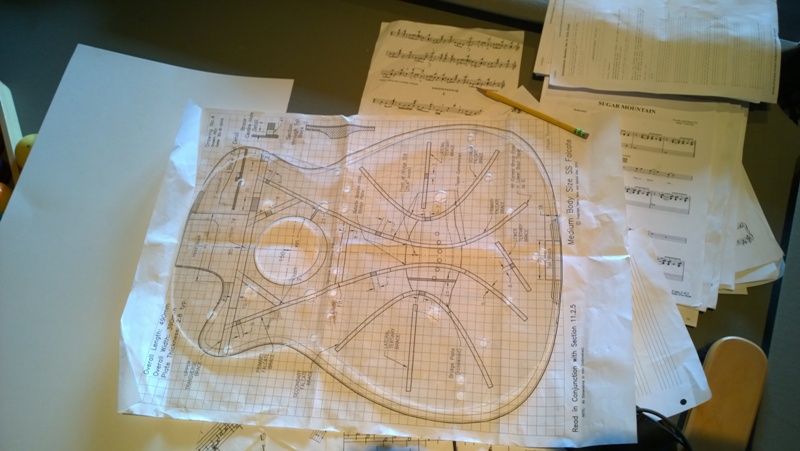
For a start I have built a Medium Sized Gore Falcate SS, its size especially the length of the body nearly matches a Hauser 37 mold and bending pattern I have. The Hauser has a narrower lower bout but still the SS falcate bracing pattern fits. Both are 12 fret to the body and the scale length are not that far off so the bridge placement is close as well. This helps as I took the time to build bending and layup molds for the braces.
From the Design book I do have some information that I can use. Looking at a couple of graphs in section 4.4 The Flexural rigidity of the Gore falcate medium sized guitar looks to be about 40 Nm^2 and the Falcate Classical is about 20. This nicely matches the 50% difference in string load between a steel string and a classical guitar.
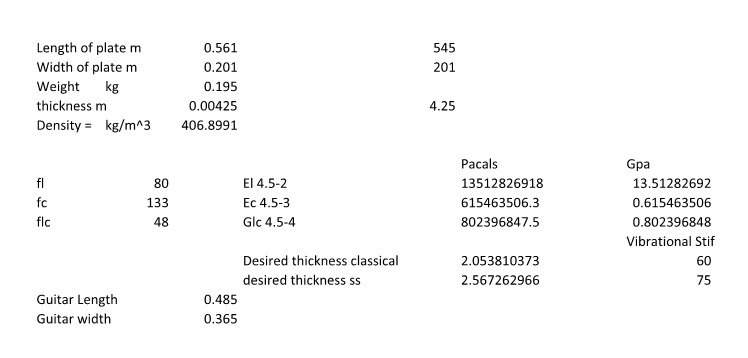
Another bit of information I happily accept for this project is using the vibrational stiffness value of 60 for a traditional classical guitar in equation 4.5-7 for plate thickness. This resulted in a 20% reduction in the top thickness when compared to a top I would build for a SS. I will use the target size from this calculation.
Assuming (I read it somewhere in the book) that the top plate is around 70% of the mass but the bracing is about 80% so the stiffness I can start making a few estimates. The 20% reduction in plate thickness while a huge change in plate stiffness (50%) results in closer to a 10% loss of stiffness to a braced top. The carbon fiber complicates doing a real Flexural rigidity calculation but given that going from SS to Classical I would be removing a half mm from a 9.5mm stack of brace and top I could see the relative loss of stiffness moving toward 15% I still have a way to go to get the Flexural rigidity halved. Also while I achieved a 10-15% reduction in stiffness with the thinner top the relative loss of mass to the top mass is about 14% (20% *.7).
My target top frequency of 190 is a bit higher than the 180 target for the SS I made, but taking into account the difference in the effect of a steel string bridge vs a classical bridge I will for this exercise ignore this difference. Given mode frequency is proportional to SQRT(k/m) where k= stiffness and m = mass), it seems that I should try to keep the k/m ratio the same. Based on my above assumption and the fact that I will remove the bridge plate and the bridge will weigh a little less, I could make the top a bit less stiff to maintain the ratio, but really within the margin of error of my estimates I could be there with just the difference is the thinner plate vs the difference in the mass of the top. I am less trusting of this comparison as I feel that the different body size and volume plus the effect of the different string load could make the comparison between the SS and the Classical more apples to oranges, still each proportional to SQRT(k/m) but from a different base frequency.
Looking at figures 4.4-9 and 4.4-12 showing the Flexural rigidity of the two guitars I am comparing , I really feel I need to lower the falcate brace heights relative to the SS a bit and just see where the top frequency ends up on this guitar. I think for a first try I will reduce the main falcate braces from 7 mm to 6 mm close to a 40% loss of stiffness in the braces. I think I may be still high (stiff) using my rough assumptions for relative flexural rigidity. But I will go with this size and see where I end up.
For a start I have built a Medium Sized Gore Falcate SS, its size especially the length of the body nearly matches a Hauser 37 mold and bending pattern I have. The Hauser has a narrower lower bout but still the SS falcate bracing pattern fits. Both are 12 fret to the body and the scale length are not that far off so the bridge placement is close as well. This helps as I took the time to build bending and layup molds for the braces.

From the Design book I do have some information that I can use. Looking at a couple of graphs in section 4.4 The Flexural rigidity of the Gore falcate medium sized guitar looks to be about 40 Nm^2 and the Falcate Classical is about 20. This nicely matches the 50% difference in string load between a steel string and a classical guitar.
Another bit of information I happily accept for this project is using the vibrational stiffness value of 60 for a traditional classical guitar in equation 4.5-7 for plate thickness. This resulted in a 20% reduction in the top thickness when compared to a top I would build for a SS. I will use the target size from this calculation.

Assuming (I read it somewhere in the book) that the top plate is around 70% of the mass but the bracing is about 80% so the stiffness I can start making a few estimates. The 20% reduction in plate thickness while a huge change in plate stiffness (50%) results in closer to a 10% loss of stiffness to a braced top. The carbon fiber complicates doing a real Flexural rigidity calculation but given that going from SS to Classical I would be removing a half mm from a 9.5mm stack of brace and top I could see the relative loss of stiffness moving toward 15% I still have a way to go to get the Flexural rigidity halved. Also while I achieved a 10-15% reduction in stiffness with the thinner top the relative loss of mass to the top mass is about 14% (20% *.7).
My target top frequency of 190 is a bit higher than the 180 target for the SS I made, but taking into account the difference in the effect of a steel string bridge vs a classical bridge I will for this exercise ignore this difference. Given mode frequency is proportional to SQRT(k/m) where k= stiffness and m = mass), it seems that I should try to keep the k/m ratio the same. Based on my above assumption and the fact that I will remove the bridge plate and the bridge will weigh a little less, I could make the top a bit less stiff to maintain the ratio, but really within the margin of error of my estimates I could be there with just the difference is the thinner plate vs the difference in the mass of the top. I am less trusting of this comparison as I feel that the different body size and volume plus the effect of the different string load could make the comparison between the SS and the Classical more apples to oranges, still each proportional to SQRT(k/m) but from a different base frequency.
Looking at figures 4.4-9 and 4.4-12 showing the Flexural rigidity of the two guitars I am comparing , I really feel I need to lower the falcate brace heights relative to the SS a bit and just see where the top frequency ends up on this guitar. I think for a first try I will reduce the main falcate braces from 7 mm to 6 mm close to a 40% loss of stiffness in the braces. I think I may be still high (stiff) using my rough assumptions for relative flexural rigidity. But I will go with this size and see where I end up.